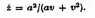Mathematics

7 NEWTON.

Quadrature of Curves

INTRODUCTION TO THE Quadrature of Curves

1. I consider mathematical quantities in this place not as consisting of very small parts; but as described by a continued motion. Lines are described, and thereby generated not by the apposition of parts, but by the continued motion of points; superficies by the motion of lines; solids by the motion of superficies; angles by the rotation of the sides; portions of time by a continual flux: and so in other quantities. These geneses really take place in the nature of things, and are daily seen in the motion of bodies. And after this manner the ancients, by drawing moveable right lines along immoveable right lines, taught the genesis of rectangles.

2. Therefore considering that quantities, which increase in equal times, and by increasing are generated, become greater or less according to the greater or less velocity with which they increase and are generated; I sought a method of determining quantities from the velocities of the motions or increments, with which they are generated; and calling these velocities of the motions or increments fluxions, and the generated quantities fluents. I fell by degrees upon the method of fluxions, which I have made use of here in the quadrature of curves, in the years 1665 and 1666.

3. Fluxions are very nearly as the augments of the fluents generated in equal but very small particles of time, and, to speak accurately, they are in the first ratio of the nascent augments; but they may be expounded by any lines which are proportional to them.

4. Thus if the areas ABC, ABDG [Fig. 1] be described by the ordinates BC, BD moving along the base AB with an uniform motion, the fluxions of these

[figure table]

Fig. 1

areas shall be to one another as the describing ordinates BC and BD, and may be expounded by these ordinates, because that these ordinates are as the nascent augments of the areas.

5. Let the ordinate BC advance from its place into any new place bc. Complete the parallelogram BC Eb, and draw the right line VTH touching the curve in C, and meeting the two lines bc and BA produced in T and V: and Bb, Ec, and Cc will be the augments now generated of the absciss AB, the ordinate BC and the curve line ACc; and the sides of the triangle GET are in the first ratio of these augments considered as nascent, therefore the fluxions of AB, BC, and AC are as the sides CE, ET, and CT of that triangle CET, and may be expounded by these same sides, or, which is the same thing, by the sides of the triangle V BC, which is similar to the triangle CET.

6. It comes to the same purpose to take the fluxions in the ultimate ratio of the evanescent parts. Draw the right line Cc, and produce it to K. Let the ordinate bc return into its former place BC, and when the points C and c coalesce, the right line CK will coincide with the tangent CH, and the evanescent triangle CEc in its ultimate form will become similar to the triangle CET, and its evanescent sides CE, Ec, and Cc will be ultimately among themselves as the sides CE, ET, and CT of the other triangle CET are, and therefore the fluxions of the lines AB, BC, and AC are in this same ratio. If the points C and c are distant from one another by any small distance, the right line CK will likewise be distant from the tangent CH by a small distance. That the right line CK may coincide with the tangent CH, and the ultimate ratios of the lines CE, Ec, and Cc may be found, the points C and c ought to coalesce and exactly coincide. The very smallest errors in mathematical matters are not to be neglected.

7. By the like way of reasoning, if a circle described with the center B and radius BC be drawn at right angles along the absciss AB, with an uniform motion, the fluxion of the generated solid A BC will be as that generating circle, and the fluxion of its superficies will be as the perimeter of that circle and the fluxion of the curve line AC jointly. For in whatever time the solid ABC is generated by drawing that circle along the length of the absciss, in the same time its superficies is generated by drawing the perimeter of that circle along the length of the curve AC. You may likewise take the following examples of this method.

8. Let the right line PB [Fig. 2], revolving about the given pole P, cut another right line AB given in position: it is required to find the proportion of the fluxions of these right lines AB and PB.

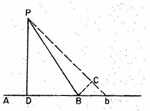Fig. 2

Let the line PB move forward from its place PB into the new place Pb. In Pb take PC equal to PB, and draw PD to AB in such manner that the angle bPD may be equal to the angle bBC; and because the triangles bBC, bPD are similar, the augment Bb will be to the augment Cb as Pb to Db. Now let Pb return into its former place PB, that these augments may evanish, then the ultimate ratio of these evanescent augments, that is the ultimate ratio of Pb to Db, shall be the same with that of PB to DB, PDB being then a right angle, and therefore the fluxion of AB is to the fluxion of PB in that same ratio.

9. Let the right line PB, revolving about the given pole P, cut other two right lines given in position, viz. AB and AE in B and E: the proportion of the fluxions of these right lines AB and AE is sought.

Let the revolving right line PB [Fig. 3] move forward from its place PB into

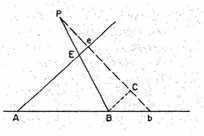Fig. 3

the new place Pb, so as to cut the lines AB, AE in the points b and e: and draw BC parallel to AE meeting Pb in C, and it will be

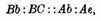
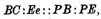
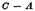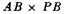
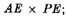
and and by joining the ratios, ¹ Now let Pb return into its former place PB, and the evanescent augment Bb will be to the evanescent augment Ee as to and therefore the fluxion of the right line AB is to the fluxion of the right line AE in the same ratio.

10. Hence if the revolving right line PB cut any curve lines given in position in the points B and E, and the right lines AB, AE now becoming moveable, touch these curves in the points of section B and E: the fluxion of the curve, which the right line AB touches, shall be to the fluxion of the curve, which the right line AE touches, as

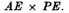
to The same thing would happen if the right line PB perpetually touched any curve given in position in the moveable point P.

11. Let the quantity x flow uniformly, and let it be proposed to find the fluxion of xⁿ.

In the same time that the quantity x, by flowing, becomes

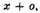
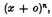
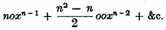
the quantity xⁿ will become that is, by the method of infinite series, And the augments o and are to one another as 1 and

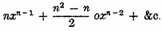
Now let these augments vanish, and their ultimate ratio wilt be 1 to

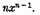
12. By like ways of reasoning, the fluxions of lines, whether right or curve in all cases, as likewise the fluxions of superficies, angles, and other quantities, may be collected by the method of prime and ultimate ratios. Now to institute an analysis after this manner in finite quantities and investigate the prime or ultimate ratios of these finite quantities when in their nascent or evanescent state, is consonant to the geometry of the ancients: and I was willing to show that, in the method of fluxions, there is no necessity of introducing figures infinitely small into geometry. Yet the analysis may be performed in any kind of figures, whether finite or infinitely small, which are imagined similar to the evanescent figures; as likewise in these figures, which, by the method of indivisibles, use to be reckoned as infinitely small, provided you proceed with due caution.

From the fluxions to find the fluents, is a much more difficult problem, and the first step of the solution is equivalent to the quadrature of curves; concerning which I wrote what follows some considerable time ago.

13. In what follows I consider indeterminate quantities as increasing or decreasing by a continued motion, that is, as flowing forwards, or backwards, and I design them by the letters z, y, x, v, and their fluxions or celerities of increasing I denote by the same letters pointed

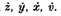
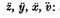
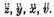
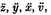
There are likewise fluxions or mutations more or less swift of these fluxions, which may be called the second fluxions of the same quantities z, y, x, v, and may be thus designed and the first fluxions of these last, or the third fluxions of z, y, x, v, are thus denoted and the fourth fluxions thus And after the same manner that are the fluxions of the quantities and these the fluxions of the quantities and these last the fluxions of the quan tities z, y, x, v: so the quantities z, y, x, v may be considered as the fluxions of others, which I shall design thus 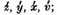and these as the fluxions of others 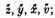and these last still as the fluxions of others Therefore &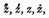c, design a series of quantities whereof every one that follows is the fluxion of the one immediately preceding, and every one that goes before, is a flowing quantity having that which immediately succeeds, for its fluxion. 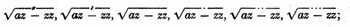The like is the series as likewise the series

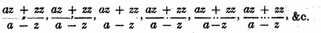
14. And it is to be remarked that any preceding quantity in these series is as the area of a curvilinear figure of which the succeeding is the rectangular ordinate, and the absciss is z: as

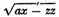
the area of a curve, whose ordinate is and absciss z. The design of all these things will appear in the following propositions.

PROPOSITION I. PROBLEM I

15. An equation being given involving any number of flowing quantities, to find the fluxions.¹

Solution. Let every term of the equation be multiplied by the index of the power² of every flowing quantity that it involves, and in every multiplication change the side or root of the power into its fluxion, and the aggregate of all the products with their proper signs, will be the new equation.

16. Explication. Let a, b, c, d, &c. be determinate and invariable quantities, and let any equation be proposed involving the flowing quantities z, y, x, &c. as

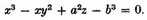
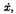
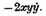
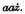
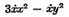
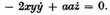
Let the terms be first multiplied by the indexes of the powers of x, and in every multiplication for the root, or x of one dimension write and the sum of the factors will be Do the same in y, and there arises Do the same in z, and there arises Let the sum of these products be put equal to nothing, and you’ll have the equation I say the relation of the fluxions is defined by this equation.

17. Demonstration. For let o be a very small quantity, and let

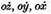
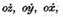
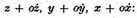
be the moments, that is the momentaneous synchronal increments of the quantities z, y, x. And if the flowing quantities are just now z, y, x, then after a moment of time, being increased by their increments these quantities shall become which being wrote in the first equation for z, y, and x, give this equation

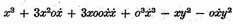
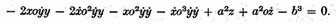
Subtract the former equation from the latter, divide the remaining equation by o, and it will be

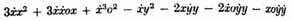
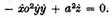
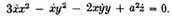
Let the quantity o be diminished infinitely, and neglecting the terms which vanish, there will remain Q.E.D.

18. A fuller explication. After the same manner if the equation were

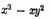
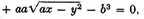
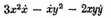
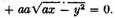
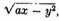
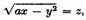
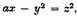
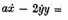
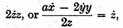
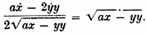
thence would be produced Where if you would take away the fluxion put and it will be and by this proposition that is And thence

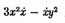
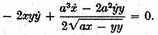
19. And by repeating the operation, you proceed to second, third, and subsequent fluxions. Let

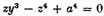
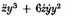
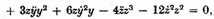
be an equation proposed, and by the first operation it becomes by the second by the third,

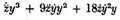
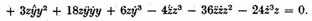
20. But when one proceeds thus to second, third, and following fluxions, it is proper to consider some quantity as flowing uniformly, and for its first fluxion to write unity, for the second and subsequent ones, nothing. Let there be given the equation

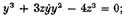
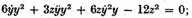
as above; and let z flow uniformly, and let its fluxion be unity: then by the first operation it shall be by the second by the third

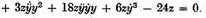
But in equations of this kind it must be conceived that the fluxions in all the terms are of the same order, i.e., either all of the first order

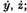
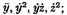
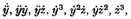
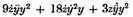
or all of the second or all of the third &c. And where the case is otherwise the order is to be completed by means of the fluxions of a quantity that flows uniformly, which fluxions are understood. Thus the last equation, by completing the third order, becomes ³

PROPOSITION II. PROBLEM II

22. To find such curves as can be squared.

Let ABC [Fig. 4] be the figure to be found, BC the rectangular ordinate, and AB the absciss. Produce CB to E, so that

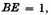
and complete the parallelogram ABED: and the fluxions of the areas ABC,ABED shall be as BC and BE. Assume therefore any equation, by which the relation of the areas may be defined, and thence the relation of the ordinates BC and BE will be given by the first proposition. Q.E.I.

The two following propositions afford examples of this.

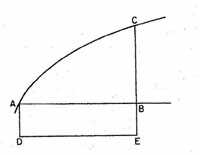Fig. 4

PROPOSITION III. THEOREM I

23. If for the absciss AB and area AE or

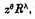
you write z promiscuously, and if for you write R: let the area of the curve be the ordinate BC shall be equal to

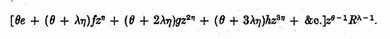⁴

24. Demonstration. For let

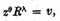
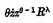
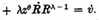
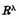
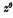
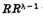
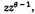
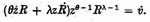
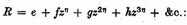
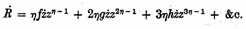
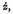
it will be (by Prop. I) For in the first term of the equation and in the second write and and it will become But it was and thence (by Prop. I) it becomes which being substituted, and BE or 1 wrote for it becomes

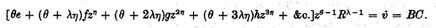
Q.E.D.

The moments of flowing quantities (that is, their indefinitely small parts, by the accession of which, in indefinitely small portions of time, they are continually increased) are as the velocities of their flowing or increasing. Wherefore, if the moment of any one, as x, be represented by the product of its celerity

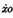
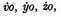
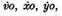
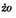
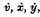
into an infinitely small quantity o (that is, by ), the moments of the others, v, y, z will be represented by because and are to each other as and

But whereas o is supposed to be indefinitely little, that it may represent the moments of quantities, the terms that are multiplied by it will be nothing m respect to the rest [termini in earn ducti pro nihilo possunt haberi cum aliis collato]; therefore I reject them, and there remains

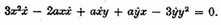
¹ This is Barrow’s notation; see Selection IV.14.

¹ Newton prefers to differentiate equations, but later also differentiates functions, often given as areas. See the remark of D’Alembert, Selection V.14, p. 343.

² [Footnote by the translator, Stewart] The word translated here power is dignitas, dignity, by which must be understood not only perfect, but also imperfect powers or surd roots, which are expressed in the manner of perfect powers, as is well known, by fractional indexes. In which sense

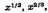
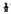
etc. are powers; and their indexes, and x the side or root. I use the word power, because dignity is seldom used in English in this sense.

³ Newton insists on homogeneity, which requires that each term of the equation has the same number of "pricks."

⁴ We have modernized Newton’s notation somewhat. He writes

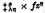
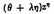
for what we writ as and instead of the square brackets he uses an overbar.

⁵ From

it follows only that the areas differ by a constant. Newton’s examples are obtained by substitutions such as in algebraic expressions.

⁶ Example:

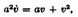
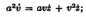
homogenized with it becomes