Authors

Topics

Events

Contents

A Source Book in Mathematics, 1200-1800

Contents:

Author: Isaac Newton | Date: 1720

Show Summary

Historical Summary

Isaac Newton was born on the day then called December 25, 1642, according to the old (Julian) calendar. The present (Gregorian) calendar was introduced (not without great opposition) into England and her American colonies in 1752, when it was decreed that September 3 should be renamed September 14, and the year, which used to begin on March 25, should henceforth begin on January 1. Those who count retroactively, therefore, call Newton’s birthday January 5, 1643, and this is the reason why the year of Newton’s birth is taken as 1642 in some accounts and 1643 in others.Newton entered Trinity College, Cambridge, in 1661, succeeded Isaac Barrow in the Lucasian professorship in Cambridge in 1669, moved to London in 1696 as Warden of the Mint, and became Master of the Mint in 1699. From 1703 till his death he was president of the Royal Society. The period from 1664 to 1669, during part of which he lived at his father’s place in Lincolnshire to escape the plague, can be referred to as his "golden period," in which he laid the foundation of his discoveries in the calculus, in mechanics, and in optics. His most famous work is the Philosophiae naturalis principia mathematica (London, 1687),which contains his celestial mechanics based on his law of universal attraction. Most of his other works were published many years after they were written. Among them is his algebra, the Arithmetica universalis, edited by William Whiston (Cambridge, 1707), written as the text of lectures between c. 1673 and c. 1683. We use here the English translation, Universal arithmetick (London), 1720). On Newton’s work see further H. W. Turnbull, The mathematical discoveries of Newton (Blackie, Glasgow, 1945).The Arithmetica contains methods of solving equations in 77 problems, investigations on the roots, including the search for divisors of polynomials, common divisors of two polynomials, and a formulation of Descartes’s sign rule. We present here a section (pp. 202–207) in which Newton deals with symmetric functions of the roots (see Selection II.6 on Girard) and then shows how to find upper limits of the roots. He uses the term "affirmative" for what we call positive, as opposed to "negative," "dimension" for our degree, "quantity" of a term for its coefficient, "rectangle" for the product of two terms, and "content" for that of more than two terms.Newton has first shown that in the equation (in our notation)-a1 is the sum of the roots, a2 "the aggregate of the rectangles of each two of the roots," and so forth. Then he continues (p. 202), writing and so on (we have somewhat modernized the spelling):

Mathematics

9 NEWTON.

The Roots of an Equation

Name: A Source Book in Mathematics, 1200-1800 - The Roots of an Equation
Author: Isaac Newton

From the generation of equations it is evident, that the known quantity of the second term of the equation, if its sign be changed, is equal to the aggregate of all the roots under their proper signs; and that of the third term, equal to the aggregate of the rectangles of each two of the roots; that of the fourth, if its sign be changed, is equal to the aggregate of the contents under each three of the roots; that of the fifth is equal to the aggregate of the contents under each four, and so on ad infinitum.

Let us assume

etc. or

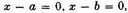
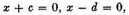
and by the continual multiplication of these we may generate equations, as above. Now, by multiplying

there will be produced the equation

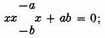
where the known quantity of the second term, if its signs are changed, viz.

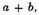
is the sum of the two roots a and b, and the known quantity of the third term is the only rectangle contained under both. Again, by multiplying this equation by

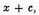
there will be produced the cubic equation

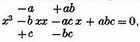
where the known quantity of the second term having its signs changed, viz.

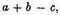
is the sum of the roots a, and b, and -c; the known quantity of the third term

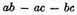
is the sum of the rectangles under each two of the roots a and b, a and -c, b and -c; and the known quantity of the fourth term under its sign changed, -abc, is the only content generated by the continual multiplication of all the roots, a by b into -c.

Moreover, by multiplying that cubic equation by

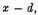
there will be produced this biquadratic one;

Where the known quantity of the second term under its signs changed, viz.

is the sum of all the roots; that of the third,

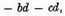
is the sum of the rectangles under every two roots; that of the fourth, its signs being changed,

is the sum of the contents under each ternary; that of the fifth, -abcd, is the only content under them all. And hence we first infer, that of any equation whose terms involve neither surds nor fractions all the rational roots, and the rectangles of any two of the roots, or the contents of any three or more of them, are some of the integral divisors of the last term;¹ and therefore when it is evident that no divisor of the last term is either a root of the equation, or rectangle, or content of two or more roots, it will also be evident that there is no root, or rectangle, or content of roots, except what is surd.

Let us suppose now, that the known quantities of the terms of any equation under their signs changed, are p, q, r, s, t, v, etc. viz. that of the second p, that of the third q, of the fourth r, of the fifth s, and so on. And the signs of the terms being rightly observed, make

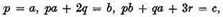
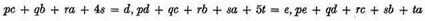
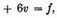² and so on in infinitum, observing the series of the progression. And a will be the sum of the roots, b the sum of the squares of each of the roots, c the sum of the cubes, d the sum of the biquadrates, e the sum of the quadrato-cubes, f the sum of the cubo-cubes, and so on. As in the equation

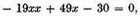
where the known quantity of the second term is -1, of the third -19, of the fourth +49, of the fifth -30; you must make

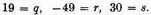
And there will thence arise

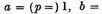
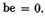
Wherefore the sum of the roots will be 1, the sum of the squares of the roots 39, the sum of the cubes -89, and the sum of the biquadrates 723, viz. the roots of that equation are 1, 2, 3, and -5, and the sum of these

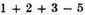
is 1; the sum of the squares,

is 39; the sum of the cubes,

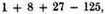
is -89; and the sum of the biquadrates,

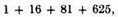
is 723.

OF THE LIMITS OF EQUATIONS.

And hence are collected the limits between which the roots of the equation shall consist, if none of them is impossible. For when the squares of all the roots are affirmative, the sum of the squares will be affirmative, and by the same argument, the sum of the biquadrates of all the roots will be greater than the bi-quadrate of the greatest root, and the sum of the cubo-cubes greater than the cubo-cube of the greatest root.

Wherefore, if you desire the limit which no roots can pass, seek the sum of the squares of the roots, and extract its square root. For this root will be greater than the greatest root of the equation. But you will come nearer the greatest root if you seek the sum of the biquadrates, and extract its biquadratic root; and yet nearer, if you seek the sum of the cubo-cubes, and extract its cubo-cubical root; and so on in infinitum.³

Thus, in the precedent equation, the square root of the sum of the squares of the roots, or

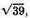
is 6½ nearly, and 6½ is farther distant from 0 than any of the roots 1, 2, 3, - 5. But the biquadratic root of the sum of the biquadrates of the roots, viz.

which is 5¼ nearly, comes nearer to the root that is most remote from nothing, viz. -5.

Rule II. If, between the sum of the squares and the sum of the biquadrates of the roots you find a mean proportional, that will be a little greater than the sum of the cubes of the roots connected under affirmative signs. And hence, the half sum of this mean proportional, and of the sum of the cubes collected under their proper signs, found as before, will be greater than the sum of the cubes of the affirmative roots, and the half difference greater than the sum of the cubes of the negative roots.⁴

And consequently, the greatest of the affirmative roots will be leas than the cube root of that half sum, and the greatest of the negative roots less than the cube root of that semi-difference.

Thus, in the precedent equation, a mean proportional between the sum of the squares of the roots 39, and the sum of the biquadrates 723, is nearly 168. The sum of the cubes under their proper signs was, as above, -89, the half sum of this and 168 is 39½, the semi-difference 128½. The cube root of the former, which is about 3½, is greater than the greatest of the affirmative roots 3. The cube root of the latter, which is

nearly, is greater than the negative root -5. By which example it may be seen how near you may come this way to the root, where there is only one negative rook or one affirmative one.

Rule III. And yet you might come nearer still, if you found a mean proportional between the sum of the biquadrates of the roots and the sum of the cubo-cubes, and if from the semi-sum and semi-difference of this, and of the sum of the quadrato-cube of the roots, you extracted the quadrato-cubical roots. For the quadrato-cubical root of the semi-sum would be greater than the greatest affirmative root, and the quadrato-cubic root of the semi-difference would be greater than the greatest negative root, but by a less excess than before. Since therefore any root, by augmenting or diminishing all the roots, may be made the least, and then the least converted into the greatest, and afterwards all besides the greatest be made negative, it is manifest how any root desired may be found nearly.

Rule IV. If all the roots except two are negative, those two may be both together found this way.

The sum of the cubes of those two roots being found aceording to the precedent method, as also the sum of the quadrato-cubes, and the sum of the quadrato-quadrato-cubes of all the roots: between the two latter sums seek a mean proportional, and that will be the difference between the sum of the cubo-cubes of the affirmative roots, and the sum of the cubo-cubes of the negative roots nearly; and consequently, the haft sum of this mean proportional, and of the sum of the cubo-cubes of all the roots, will be the sum of the cubo-cubes of the affirmative roots, and the semi-difference will be the sum of the cubo-cubes of the negative roots. Having therefore both the sum of the cubes, and also the sum of the cubo-cubes of the two affirmative roots, from the double of the latter sum subtract the square of the former sum, and the square root of the remainder will he the difference of the cubes of the two roots. And having both the sum and difference of the cubes, you will have the cubes themselves. Extract their cube roots, and you will nearly have the two affirmative roots of the equation. And if in higher powers you should do the like, you will have the roots yet more nearly. But these limitations, by reason of the difficulty of the calculus, are of less use, and extend only to those equations that have no imaginary roots. Wherefore I will now shew how to find the limits another way, which is more easy, and extends to all equations.

Rule V. Multiply every term of the equation by the number of its dimensions, and divide the product by the root of the equation. Then again multiply every one of the terms that come out by a number less by unity than before, and divide the product by the root of the equation. And so go on, by always multiplying by numbers less by unity than before, and dividing the product by the root, till at length all the terms are destroyed, whose signs are different from the sign of the first or highest term, except the last. And that number will be greater than any affirmative root; which being writ in the terms that come out for the root, makes the aggregate of those

which were each time produced by multiplication to have always the same sign with the first or highest term of the equation.⁵

As if there was proposed the equation

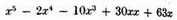
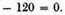
I first multiply this thus;

Then again multiply the terms that come out dividcd by x, thus;

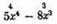
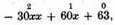
and dividing the terms that come out again by x, there comes out

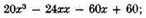
which, to lessen them, I divide by the greatest common divisor 4, and you have

These being again multiplied by the progression 3, 2, 1, 0, and divided by x, become

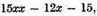
and again divided by 3 become

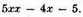
And these multiplied by the progression 2, 1, 0, and divided by 2x become

Now, since the highest term of the equation x⁵ is affirmative, I try what number writ in these producta for x will cause them all to be affirmative. And by trying 1, you have

affirmative; but

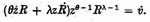
you have -4 negative. Wherefore the limit will be greater than 1. I therefore try some greater number, as 2. And substituting 2 in each for x, they become

Wherefore, since the numbers that come out 8.7.1.79.46. are all affirmative, the number 2 will be greater than the greatest of the affirmative roots. In like manner, if I would find the limit of the negative roots, I try negative numbers. Or that which is all one, I change the signs of every other term, and try affirmative ones. But having changed the signs of every other term, the quantities in which the numbers are to be substituted, will become

Out of these I choose some quantity wherein the negative terms seem most prevalent; suppose

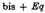
and here substituting for x the numbers 1 and 2, there come out the negative numbers -14 and -33. Whence the limit will be greater than -2. But substituting the number 3, there comes out the affirmative number 234. And in like manner in the other quantities, by substituting the number 3 for x, there comes out always an affirmative number, which may be seen by bare inspection. Wherefore the number -3 is greater than all the negative roots. And so you have the limits 2 and -3, between which are all the roots.

¹ This sentence has been translated from the original Latin text, since the English text is garbled. The term surd is usually used for incommensurable roots of a commensurable number, as

but also means any nonrational number; "quantities partly rationall and partly surde," writes Recorde in his Pathewaie to knowledge (London, 1551). The term, meaning deaf or mute, is a Latin translation of an Arabic translation of the Greek term alogos, meaning irrational.

² These sums are known as sums of Newton, although they were known to Girard (Selection II.6). The general formulas for the equation

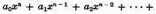
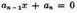
are

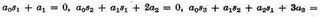
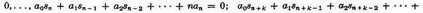
for

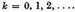
Here s_i is the sum of the ith powers of the roots. They go in essential back to C. Maclaurin, A treatise of algebra (London, 1748), Part II, 141–143.

³ If x_μ is the largest root in absolute value, then for any positive integer

and

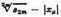
will tend to zero with increasing m. The proof follows from the fact that 8_2m is always positive.

⁴ Let

where

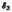
is the sum of the third powers of the positive roots, and

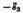
that of the negative roots. Then

⁵ This rule is known as Newton’s rule, and can be expressed in modern language as follows: If

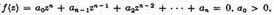
then every number

for which f(z) and its derivatives

are positive is an upper limit for the positive roots of

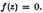
The rule can be proved as a corollary to the theorem of Fourier (1831; also called after Budan); see, for instance W. S. Burnside and A. W. Panton, The theory of equations (3rd ed.; Hedges, Figgis and Co., Dublin, 1892), 170, 182.

Contents:

Related Resources

Sir Isaac Newton

Download Options

Title: A Source Book in Mathematics, 1200-1800

Select an option:

*Note: A download may not start for up to 60 seconds.

Email Options

Title: A Source Book in Mathematics, 1200-1800

Select an option:

Email addres:

*Note: It may take up to 60 seconds for for the email to be generated.

Chicago: Isaac Newton, "The Roots of an Equation," A Source Book in Mathematics, 1200-1800, ed. William Whiston in A Source Book in Mathematics, 1200-1800, ed. D. J. Struik (Princeton: Princeton University Press, 1969, 1986), 93–99. Original Sources, accessed April 24, 2024, http://originalsources.com/Document.aspx?DocID=9LY69ZXXJ7KCBKS.

MLA: Newton, Isaac. "The Roots of an Equation." A Source Book in Mathematics, 1200-1800, edited by William Whiston, in A Source Book in Mathematics, 1200-1800, edited by D. J. Struik, Princeton, Princeton University Press, 1969, 1986, pp. 93–99. Original Sources. 24 Apr. 2024. http://originalsources.com/Document.aspx?DocID=9LY69ZXXJ7KCBKS.

Harvard: Newton, I, 'The Roots of an Equation' in A Source Book in Mathematics, 1200-1800, ed. . cited in 1969, 1986, A Source Book in Mathematics, 1200-1800, ed. , Princeton University Press, Princeton, pp.93–99. Original Sources, retrieved 24 April 2024, from http://originalsources.com/Document.aspx?DocID=9LY69ZXXJ7KCBKS.