13.

HEREDITARY GENIUS¹

ByFRANCISn/aGALTONn/an/an/an/a

I propose to show in this book that a man’s natural abilities are derived by inheritance, under exactly the same limitations as are the form and physical features of the whole organic world. Consequently, as it is easy, notwithstanding those limitations, to obtain by careful selection a permanent breed of dogs or horses gifted with peculiar powers of running, or of doing anything else, so it would be quite practicable to produce a highly-gifted race of men by judicious marriages during several consecutive generations. I shall show that social agencies of an ordinary character, whose influences are little suspected, are at this moment working towards the degradation of human nature, and that others are working towards its improvement. I conclude that each generation has enormous power over the natural gifts of those that follow, and maintain that it is a duty we owe to humanity to investigate the range of that power, and to exercise it in a way that, without being unwise towards ourselves, shall be most advantageous to future inhabitants of the earth. . . .

The general plan of my argument is to show that high reputation is a pretty accurate test of high ability; next to discuss the relationships of a large body of fairly eminent men—namely, the Judges of England from 1660 to 1868, the Statesmen of the time of George III, and the Premiers during the last 100 years—and to obtain from these a general survey of the laws of heredity in respect to genius. Then I shall examine, in order, the kindred of the most illustrious Commanders, men of Literature and of Science, Poets, Painters, and Musicians, of whom history speaks. I shall also discuss the kindred of a certain selection of Divines and of modern Scholars. Then will follow a short chapter, by way of comparison, on the hereditary transmission of physical gifts, as deduced from the relationships of certain classes of Oarsmen and Wrestlers. Lastly, I shall collate my results, and draw conclusions. . . .

CLASSIFICATION OF MEN ACCORDING TO THEIR REPUTATION

The arguments by which I endeavour to prove that genius is hereditary, consist in showing how large is the number of instances in which men who are more or less illustrious have eminent kinsfolk. . . .

I look upon social and professional life as a continuous examination. All are candidates for the good opinions of others, and for success in their several professions, and they achieve success in proportion as the general estimate is large of their aggregate merits. In ordinary scholastic examiations marks are allotted in stated proportions to various specified subjects—so many for Latin, so many for Greek, so many for English history, and the rest. The world, in the same way, but almost unconsciously, allots marks to men. It gives them for originality of conception, for enterprise, for activity and energy, for administrative skill for various acquirements, for power of literary expression, for oratory, and much besides of general value, as well as for more specially professional merits. It does not allot these marks according to a proportion that can easily be stated in words, but there is a rough common-sense that governs its practice with a fair approximation to constancy. Those who have gained most of these tacit marks are ranked, by the common judgment of the leaders of opinion, as the foremost men of their day.

The metaphor of an examination may be stretched much further. As there are alternative groups in any one of which a candidate may obtain honours, so it is with reputations—they may be made in law, literature, science, art, and in a host of other pursuits. Again: as the mere attainment of a general fair level will obtain no honours in an examination, no more will it do so in the struggle for eminence. A man must show conspicuous power, in at least one subject in order to achieve a high reputation.

Let us see how the world classifies people, after examining each of them, in her patient, persistent manner, during the years of their manhood. How many men of "eminence" are there, and what proportion do they bear to the whole community?

I will begin by analyzing a very painstaking biographical handbook, lately published by Routledge and Co., called "Men of the Time." Its intention, which is very fairly and honestly carried out, is to include none but those whom the world honours for their ability. The catalogue of names is 2,500, and a full half of it consists of American and Continental celebrities. . . .

On looking over the book, I am surprised to find how large a proportion of the "Men of the Time" are past middle age. It appears that in the cases of high (but by no means in that of the highest) merit, a man must outlive the age of fifty to be sure of being widely appreciated. It takes time for an able man, born in the humbler ranks of life, to emerge from them and to take his natural position. It would not, therefore, be just to compare the numbers of Englishmen in the book with that of the whole adult male population of the British Isles; but it is necessary to confine our examination to those of the celebrities who are past fifty years of age, and to compare their number with that of the whole male population who are also above fifty years. I estimate, from examining a large part of the book, that there are about 850 of these men, and that 500 of them are decidedly well known to persons familiar with literary and scientific society. Now, there are about two millions of adult males in the British Isles above fifty years of age; consequently, the total number of the "Men of the Time" are as 425 to a million, and the more select part of them as 250 to a million. . . .

Another estimate of the proportion of eminent men to the whole population was made on a different basis, and gave much the same result. I took the obituary of the year 1868, published in the Times on January 1st, 1869, and found in it about fifty names of men of the more select class. This was in one sense a broader, and in another a more rigorous selection than that which I have just described. It was broader, because I included the names of many whose abilities were high, but who died too young to have earned the wide reputation they deserved; and it was more rigorous, because I excluded old men who had earned distinction in years gone by, but had not shown themselves capable in later times to come again to the front. On the first ground, it was necessary to lower the limit of the age of the population with whom they should be compared. Forty-five years of age seemed to me a fair limit, including, as it was supposed to do, a year or two of broken health preceding decease. Now, 210,000 males die annually in the British Isles above the age of forty-five; therefore, the ratio of the more select portion of the Men of the Time on these data is as 50 to 210,000, or as 238 to a million.

Thirdly, I consulted obituaries of many years back, when the population of these islands was much smaller, and they appeared to me to lead to similar conclusions, viz., that 250 to a million is an ample estimate. . . .

These considerations define the sense in which I propose to employ the word "eminent." When I speak of an eminent man, I mean one who has achieved a position that is attained by only 250 persons in each million of men, or by one person in each 4,000. 4,000 is a very large number—difficult for persons to realize who are not accustomed to deal with great assemblages. On the most brilliant of starlight nights there are never so many as 4,000 stars visible to the naked eye at the same time; yet we feel it to be an extraordinary distinction to a star to be accounted as the brightest in the sky. This, be it remembered, is my narrowest area of selection. I propose to introduce no name whatever into my lists of kinsmen (unless it be marked off from the rest by brackets that is less distinguished. . . .

CLASSIFICATION OF MEN ACCORDING TO THEIR NATURAL GIFTS

I have no patience with the hypothesis occasionally expressed, and often implied, especially in tales written to teach children to be good, that babies are born pretty much alike, and that the sole agencies in creating differences between boy and boy, and man and man, are steady application and moral effort. It is in the most unqualified manner that I object to pretensions of natural equality. The experiences of the nursery, the school, the University, and of professional careers, are a chain of proofs to the contrary. I acknowledge freely the great power of education and social influences in developing the active powers of the mind, just as I acknowledge the effect of use in developing the muscles of a blacksmith’s arm, and no further. Let the blacksmith labour as he will, he will find that there are certain feats beyond his power that are well within the strength of a man of herculean make, even although the latter may have led a sedentary life. Some years ago, the Highlanders held a grand gathering in Holland Park, where they challenged all England to compete with them in their games of strength. The challenge was accepted, and the well-trained men of the hills were beaten in the foot-race by a youth who was stated to be a pure Cockney, the clerk of a London banker.

Everybody who has trained himself to physical exercises discovers the extent of his muscular powers to a nicety. When he begins to walk, to row, to use the dumb bells, or to run, he finds to his great delight that his thews strengthen, and his endurance of fatigue increases day after day. So long as he is a novice, he perhaps flatters himself there is hardly an assignable limit to the education of his muscles; but the daily gain is soon discovered to diminish, and at last it vanishes altogether. His maximum performance becomes a rigidly determinate quantity. He learns to an inch, how high or how far he can jump, when he has attained the highest state of training. He learns to half a pound, the force he can exert on the dynamometer, by compressing it. He can strike a blow against the machine used to measure impact, and drive its index to a certain graduation, but no further. So it is in running, in rowing, in walking, and in every other form of physical exertion. There is a definite limit to the muscular powers of every man, which he cannot by any education or exertion overpass.

This is precisely analogous to the experience that every student has had of the working of his mental powers. The eager boy, when he first goes to school and confronts intellectual difficulties, is astonished at his progress. He glories in his newly-developed mental grip and growing capacity for application, and, it may be, fondly believes it to be within his reach to become one of the heroes who have left their mark upon the history of the world. The years go by; he competes in the examinations of school and college, over and over again with his fellows, and soon finds his place among them. He knows he can beat such and such of his competitors; that there are some with whom he runs on equal terms, and others whose intellectual feats he cannot even approach. Probably his vanity still continues to tempt him, by whispering in a new strain. It tells him that classics, mathematics, and other subjects taught in universities, are mere scholastic specialties, and no test of the more valuable intellectual powers. It reminds him of numerous instances of persons who had been unsuccessful in the competitions of youth, but who had shown powers in after-life that made them the foremost men of their age. Accordingly, with newly furbished hopes, and with all the ambition of twenty-two years of age, he leaves his University and enters a larger field of competition. The same kind of experience awaits him here that he has already gone through. Opportunities occur—they occur to every man—and he finds himself incapable of grasping them. He tries, and is tried in many things. In a few years more, unless he is incurably blinded by self-conceit, he learns precisely of what performances he is capable, and what other enterprises lie beyond his compass. When he reaches mature life, he is confident only within certain limits, and knows, or ought to know, himself just as he is probably judged of by the world, with all his unmistakable weakness and all his undeniable strength. He is no longer tormented into hopeless efforts by the fallacious promptings of overweening vanity, but he limits his undertakings to matters below the level of his reach, and finds true moral repose in an honest conviction that he is engaged in as much good work as his nature has rendered him capable of performing. . . .

To conclude, the range of mental power between—I will not say the highest Caucasian and the lowest savage—but between the greatest and least of English intellects, is enormous. There is a continuity of natural ability reaching from one knows not what height, and descending to one can hardly say what depth. I propose in this chapter to range men according to their natural abilities, putting them into classes separated by equal degrees of merit, and to show the relative number of individuals included in the several classes. Perhaps some persons might be inclined to make an offhand guess that the number of men included in the several classes would be pretty equal. If he thinks so, I can assure him he is most egregiously mistaken.

The method I shall employ for discovering all this, is an application of the very curious theoretical law of "deviation from an average." First, I will explain the law, and then I will show that the production of natural intellectual gifts comes justly within its scope.

The law is an exceedingly general one. M. Quetelet, the Astronomer-Royal of Belgium, and the greatest authority on vital and social statistics, has largely used it in his inquiries. He has also constructed numerical tables, by which the necessary calculations can be easily made, whenever it is desired to have recourse to the law. . . .

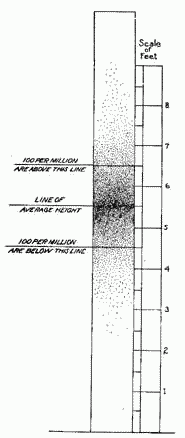
So much has been published in recent years about statistical deductions, that I am sure the reader will be prepared to assent freely to the following hypothetical case: Suppose a large island inhabited by a single race who intermarried freely, and who had lived for many generations under constant conditions; then the average height of the male adults of that population would undoubtedly be the same year after year. Also—still arguing from the experience of modern statistics, which are found to give constant results in far less carefully-guarded examples—we should undoubtedly find, year after year, the same proportion maintained between the number of men of different heights. I mean, if the average stature was found to be sixty-six inches, and if it was also found in any one year that 100 per million exceeded seventy-eight inches, the same proportion of 100 per million would be closely maintained in all other years. An equal constancy of proportion would be maintained between any other limits of height we pleased to specify, as between seventy-one and seventy-two inches; between seventy-two and seventy-three inches; and so on. Statistical experiences are so invariably confirmatory of what I have stated would probably be the case, as to make it unnecessary to describe analogous instances. Now, at this point, the law of deviation from an average steps in. It shows that the number per million whose heights range between seventy-one and seventy-two inches (or between any other limits we please to name) can be predicted from the previous datum of the average, and of any one other fact, such as that of 100 per million exceeding seventy-eight inches.

The diagram will make this more intelligible. Suppose a million of the men to stand in turns, with their backs against a vertical board of sufficient height, and their heights to be dotted off upon it. The board would then present the appearance shown in the diagram. The line of average height is that which divides the dots into two equal parts, and stands, in the case we have assumed, at the height of sixty-six inches. The dots will be found to be ranged so symmetrically on either side of the line of average, that the lower half of the diagram will be almost a precise reflection of the upper. Next, let a hundred dots be counted from above downwards, and let a line be drawn below them. According to the conditions, this line will stand at the height of seventy-eight inches. Using the data afforded by these two lines, it is possible, by the help of the law of deviation from an average, to reproduce, with extraordinary closeness, the entire system of dots on the board.

M. Quetelet gives tables in which the uppermost line, instead of cutting off 100 in a million, cuts off only one in a million. He divides the intervals between that line and the line of average, into eighty equal divisions, and gives the number of dots that fall within each of those divisions. It is easy, by the help of his tables, to calculate what would occur under any other system of classification we pleased to adopt.

This law of deviation from an average is perfectly general in its application. Thus, if the marks had been made by bullets fired at a horizontal line stretched in front of the target, they would have been distributed according to the same law. Wherever there is a large number of similar events, each due to the resultant influences of the same variable conditions, two effects will follow. First, the average value of those events will be constant; and, secondly, the deviations of the several events from the average, will be governed by this law (which is, in principle, the same as that which governs runs of luck at a gaming-table). . . .

I selected the hypothetical case of a race of men living on an island and freely intermarrying, to ensure the conditions under which they were all supposed to live, being uniform in character. It will now be my aim to show there is sufficient uniformity in the inhabitants of the British Isles to bring them fairly within the grasp of this law.

For this purpose, I first call attention to an example given in Quetelet’s book. It is of the measurements of the circumferences of the chests of a large number of Scotch soldiers. The Scotch are by no means a strictly uniform race, nor are they exposed to identical conditions. They are a mixture of Celts, Danes, Anglo-Saxons, and others, in various proportions, the Highlanders being almost purely Celts. On the other hand, these races, though diverse in origin, are not very dissimilar in character. Consequently, it will be found that their deviations from the average, follow theoretical computations with remarkable accuracy. The instance is as follows: M. Quetelet obtained his facts from the thirteenth volume of the Edinburgh Medical Journal, where the measurements are given in respect to 5,738 soldiers, the results being grouped in order of magnitude, proceeding by differences of one inch. Professor Quetelet compares these results with those that his tables give, and here is the result. The marvellous accordance between fact and theory must strike the most unpractised eye.

TABLE I SCOTCH SOLDIERS

I should say that, for the sake of convenience, both the measurements and calculations have been reduced to per ten thousands.

I argue from the results obtained from Frenchmen and from Scotchmen, that, if we had measurements of the adult males in the British Isles, we should find those measurements to range in close accordance with the law of deviation from an average, although our population is as much mingled as I described that of Scotland to have been, and although Ireland is mainly peopled with Celts. Now, if this be the case with stature, then it will be true as regards every other physical feature—as circumference of head, size of brain, weight of grey matter, number of brain fibres, etc.; and thence, by a step on which no physiologist will hesitate, as regards mental capacity.

This is what I am driving at—the analogy clearly shows there must be a fairly constant average mental capacity in the inhabitants of the British Isles, and that the deviations from that average—upwards towards genius, and downwards towards stupidity—must follow the law that governs deviations from all true averages. . . .

The number of grades into which we may divide ability is purely a matter of option. We may consult our convenience by sorting Englishmen into a few large classes, or into many small ones. I will select a system of classification that shall be easily comparable with the numbers of eminent men, as determined in the previous chapter. We have seen that 250 men per million become eminent; accordingly, I have so contrived the classes in the following table with the two highest, F and G, together with X (which includes all cases beyond G, and which are unclassed), shall amount to about that number—namely, to 248 per million.

It will, I trust, be clearly understood that the numbers of men in the several classes in my table depend on no uncertain hypothesis. They are determined by the assured law of deviations from an average. It is an absolute fact that if we pick out of each million the one man who is naturally the ablest, and also the one man who is the most stupid, and divide the remaining 999,998 men into fourteen classes, the average ability in each being separated from that of its neighbours by equal grades, then the numbers in each of those classes will, on the average of many millions, be as is stated in the table. The table may be applied to special, just as truly as to general ability. It would be true for every examination that brought out natural gifts, whether held in painting, in music, or in statesmanship. The proportions between the different classes would be made up of different individuals, according as the examination differed in its purport.

It will be seen that more than half of each milion is contained in the two mediocre classes a and A; the four mediocre classes a, b, A, B, contain

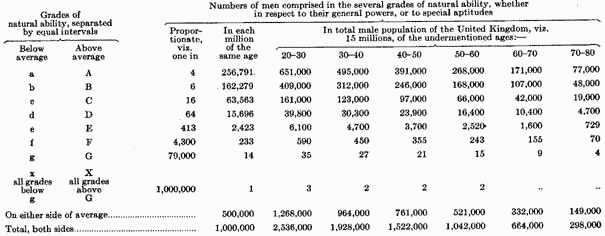TABLE II CLASSIFICATION OF MEN ACCORDING TO THEIR NATURAL GIFTS

more than four-fifths, and the six mediocre classes more than nineteen-twentieths of the entire population. Thus, the rarity of commanding ability, and the vast abundance of mediocrity, is no accident, but follows of necessity, from the very nature of these things. . . .

The class C possesses abilities a trifle higher than those commonly possessed by the foreman of an ordinary jury. D includes the mass of men who obtain the ordinary prizes of life. E is a stage higher. Then we reach F, the lowest of those yet superior classes of intellect, with which this volume is chiefly concerned. . . .

THE JUDGES OF ENGLAND BETWEEN 1660 AND 1865

The Judges of England, since the restoration of the monarchy in 1660, form a group peculiarly well adapted to afford a general outline of the extent and limitations of heredity in respect to genius. A judgeship is a guarantee of its possessor being gifted with exceptional ability; the Judges are sufficiently numerous and prolific to form an adequate basis for statistical inductions, and they are the subjects of several excellent biographical treatises. It is therefore well to begin our inquiries with a discussion of their relationships. We shall quickly arrive at definite results, which subsequent chapters, treating of more illustrious men, and in other careers, will check and amplify.

It is necessary that I should first say something in support of my assertion, that the office of a judge is really a sufficient guarantee that its possessor is exceptionally gifted. In other countries it may be different to what it is with us, but we all know that in England, the Bench is never spoken of without reverence for the intellectual power of its occupiers. A seat on the Bench is a great prize, to be won by the best men. . . .

If not always the foremost, the Judges are therefore among the foremost, of a vast body of legal men. . . .

There are 286 judges within the limits of my inquiry; 109 of them have one or more eminent relations, and three others have relations whom I have noticed, but they are marked off with brackets, and are therefore not to be included in the following statistical deductions. . . .

First, it will be observed, that the judges are so largely interrelated, that 109 of them are grouped into only 85 families. There are seventeen doublets, among the judges, two triplets, and one quadruplet. In addition to these, might be counted six other sets, consisting of those whose ancestors sat on the Bench previously to the accession of Charles II, namely, Bedingfield, Forster, Hyde, Finch, Windham, and Lyttleton. Another fact to be observed, is the nearness of the relationships in my list. The single letters are far the most common. Also, though a man has twice as many grandfathers as fathers, and probably more than twice

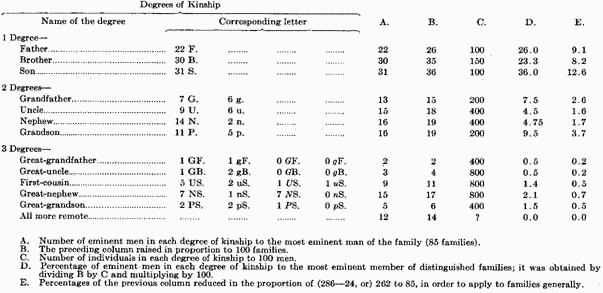TABLE III

as many grandsons as sons, yet the Judges are found more frequently to have eminent fathers than grandfathers, and eminent sons than grandsons. In the third degree of relationship, the eminent kinsmen are yet more rare, although the number of individuals in those degrees is increased in a duplicate proportion. When a judge has no more than one eminent relation, that relation is nearly always to be found in the first or second degree. . . . I annex a table (Table III) . . . which exhibits these facts with great clearness. Column A contains the facts just as they were observed, and column D shows the percentage of individuals, in each degree of kinship to every 100 judges, who have become eminent.

What I profess to prove is this: that if two children are taken, of whom one has a parent exceptionally gifted in a high degree—say as one in 4,000, or as one in a million—and the other has not, the former child has an enormously greater chance of turning out to be gifted in a high degree, than the other. Also, I argue that, as a new race can be obtained in animals and plants, and can be raised to so great a degree of purity that it will maintain itself, with moderate care in preventing the more faulty members of the flock from breeding, so a race of gifted men might be obtained, under exactly similar conditions. . . .

COMPARISON OF RESULTS

Let us now bring our scattered results side to side, for the purpose of comparison, and judge of the extent to which they corroborate one another,—how far they confirm the provisional calculations made in the chapter on Judges from more scanty data, and where and why they contrast.

The number of cases of hereditary genius analysed in the several chapters of my book amounts to a large total. I have dealt with no less than 300 families containing between them nearly 1,000 eminent men, of whom 415 are illustrious, or, at all events, of such note as to deserve being printed in black type at the head of a paragraph. If there be such a thing as a decided law of distribution of genius in families, it is sure to become manifest when we deal statistically with so large a body of examples.

In comparing the results obtained from the different groups of eminent men, it will be our most convenient course to compare the columns B of the several tables. Column B gives the number of kinsmen in various degrees, on the supposition that the number of families in the group to which it refers is 100. All the entries under B have therefore the same common measure, they are all percentages, and admit of direct intercomparison. I hope I have made myself quite clear: lest there should remain any misapprehension, it is better to give an example. Thus, the families of Divines are only 25 in number, and in those 25 families there are 7

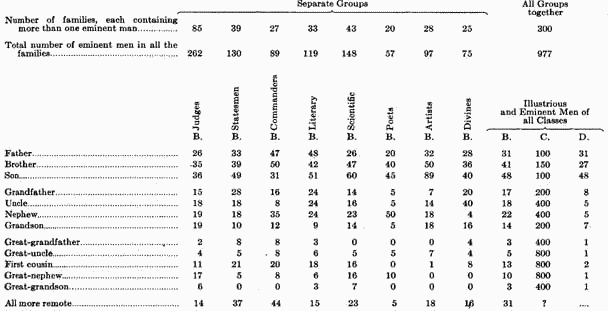TABLE IV

eminent fathers, 9 brothers, and 10 sons; now in order to raise these numbers to percentages, 7, 9, and 10 must be multiplied by the number of times that 25 goes into 100, namely by 4. They will then become 28, 36, and 40. . . .

The general uniformity in the distribution of ability among the kinsmen in the different groups, is strikingly manifest. The eminent sons are almost invariably more numerous than the eminent fathers. On proceeding further down the table, we come to a sudden dropping off of the numbers at the second grade of kinship, namely, at the grandfathers, uncles, nephews, and grandsons: this diminution is conspicuous in the entries in column D, the meaning of which has already been described. On reaching the third grade of kinship, another abrupt dropping off in numbers is again met with, but the first cousins are found to occupy a decidedly better position than other relations within the third grade. . . .

I reckon the chances of kinsmen of illustrious men rising, or having risen, to be 15½ to 100 in the case of fathers, 13½ to 100 in the case of brothers, 24 to 100 in the case of sons. Or, putting these and the remaining proportions into a more convenient form, we obtain the following results. In first grade: the chance of the father is 1 to 6; of each brother, 1 to 7; of each son, 1 to 4. In second grade: of each grandfather, 1 to 25; of each uncle, 1 to 40; of each nephew, 1 to 40; of each grandson, 1 to 29. In the third grade, the chance of each member is about 1 to 200, excepting in the case of first cousins, where it is 1 to 100. . . .

THE COMPARATIVE WORTH OF DIFFERENT RACES

I have now completed what I have to say concerning the kinships of individuals, and proceed, in this chapter, to attempt a wider treatment of my subject, through a consideration of nations and races. . . .

Let us, then, compare the negro race with the Anglo-Saxon, with respect to those qualities alone which are capable of producing judges, statesmen, commanders, men of literature and science, poets, artists, and divines. If the negro race in America had been affected by no social disabilities, a comparison of their achievements with those of the whites in their several branches of intellectual effort, having regard to the total number of their respective populations, would give the necessary information. As matters stand, we must be content with much rougher data.

First, the negro race has occasionally, but very rarely, produced such men as Toussaint l’Ouverture, who are of our class F; that is to say, its X, or its total classes above G, appear to correspond with our F, showing a difference of not less than two grades between the black and white races, and it may be more.

Secondly, the negro race is by no means wholly deficient in men capable of becoming good factors, thriving merchants, and otherwise considerably raised above the average of whites—that is to say, it can not infrequently supply men corresponding to our class C, or even D. It will be recollected that C implies a selection of 1 in 16, or somewhat more than the natural abilities possessed by average foremen of common juries, and that D is as 1 in 64—a degree of ability that is sure to make a man successful in life. In short, classes E and F, of the negro may roughly be considered as the equivalent of our C and D—a result which again points to the conclusion, that the average intellectual standard of the negro race is some two grades below our own.

Thirdly, we may compare, but with much caution, the relative position of negroes in their native country with that of the travellers who visit them. The latter, no doubt, bring with them the knowledge current in civilized lands, but that is an advantage of less importance than we are apt to suppose. A native chief has as good an education in the art of ruling men, as can be desired; he is continually exercised in personal government, and usually maintains his place by the ascendancy of his character, shown every day over his subjects and rivals. A traveller in wild countries also fills, to a certain degree, the position of a commander, and has to confront native chiefs at every inhabited place. The result is familiar enough—the white traveller almost invariably holds his own in their presence. It is seldom that we hear of a white traveller meeting with a black chief whom he feels to be the better man. I have often discussed this subject with competent persons, and can only recall a few cases of the inferiority of the white man,—certainly not more than might be ascribed to an average actual difference of three grades, of which one may be due to the relative demerits of native education, and the remaining two to a difference in natural gifts. . . .

The ablest race of whom history bears record is unquestionably the ancient Greek, partly because their master-pieces in the principal departments of intellectual activity are still unsurpassed, and in many respects unequalled, and partly because the population that gave birth to the creators of those master-pieces was very small. Of the various Greek sub-races, that of Attica was the ablest, and she was no doubt largely indebted to the following cause, for her superiority. Athens opened her arms to immigrants, but not indiscriminately, for her social life was such that none but very able men could take any pleasure in it; on the other hand, she offered attractions such as men of the highest ability and culture could find in no other city. Thus, by a system of partly unconscious selection, she built up a magnificent breed of human animals, which, in the space of one century—viz., between 530 and 430 B.C.—produced the following illustrious persons, fourteen in number:

Statesmen and Commanders.—Themistocles (mother an alien), Miltiades, Aristeides, Cimon (son of Miltiades), Pericles (son of Xanthippus, the victor at Mycale).

Literary and Scientific Men.—Thucydides, Socrates, Xenophon, Plato.

Poets.—Æschylus, Sophocles, Euripides, Aristophanes.

Sculptor.—Phidias.

We are able to make a closely-approximate estimate of the population that produced these men, because the number of the inhabitants of Attica has been a matter of frequent inquiry, and critics appear at length to be quite agreed in the general results. It seems that the little district of Attica contained, during its most flourishing period (Smith’s Class. Geog. Dict.), less than 90,000 native free-born persons, 40,000 resident aliens, and a labouring and artisan population of 400,000 slaves. The first item is the only one that concerns us here, namely, the 90,000 free-born persons. Again, the common estimate that population renews itself three times in a century is very close to the truth, and may be accepted in the present case. Consequently, we have to deal with a total population of 270,000 free-born persons, or 135,000 males, born in the century I have named. Of these, about one-half, or 67,500, would survive the age of 26, and one-third, or 45,000, would survive that of 50. As 14 Athenians became illustrious, the selection is only 1 to 4,822 in respect to the former limitation, and as 1 to 3,214 in respect to the latter. Referring to the table on page 143, it will be seen that this degree of selection corresponds very fairly to the classes F (1 in 4,300) and above, of the Athenian race. Again, as G is one-sixteenth or one-seventeenth as numerous as F, it would be reasonable to expect to find one of class G among the fourteen; we might, however, by accident, meet with two, three, or even four of that class—say Pericles, Socrates, Plato, and Phidias.

Now let us attempt to compare the Athenian standard of ability with that of our own race and time. We have no men to put by the side of Socrates and Phidias, because the millions of all Europe, breeding as they have done for the subsequent 2,000 years, have never produced their equals. They are, therefore, two or three grades above our G—they might rank as I or J. But, supposing we do not count them at all, saying that some freak of nature acting at that time, may have produced them, what must we say about the rest? Pericles and Plato would rank, I suppose, the one among the greatest of philosophical statesmen, and the other as at least the equal of Lord Bacon. They would, therefore stand somewhere among our unclassed X, one or two grades above G—let us call them between H and I. All the remainder—the F of the Athenian race—would rank above our G, and equal to or close upon our H. It follows from all this, that the average ability of the Athenian race is, on the lowest possible estimate, very nearly two grades higher than our own—that is, about as much as our race is above that of the African negro.

¹