Mathematics

Jacobi on the Jacobi Theta Functions

¹

In my work Fundamenta nova theoriae functionum ellipticarum, proceeding from the consideration of elliptic integrals, I arrived at the curious series which I denote by the symbols Θ and H, and which form the numerators and denominators of the elliptic functions sin am u, cos am u, Δ am u.

Reversing the historic path of discovery of the elliptic functions, I plan to go the opposite way below. Without assuming anything from the theory of elliptic functions, proceeding from the series Θ and H and using a simple principle, I shall establish the relations which these series satisfy. From these relations I shall derive an addition theorem for the quotients of the series, and from this, the differential formulas which lead directly to the elliptic integrals.

1.

The doubly infinite series which form the point of departure for our study have as their general term exponentials with quadratic exponent . . .

² where the summation extends over all positive and negative integers r. Of the three coefficients a, b, and c, the last can be set equal to zero without loss of generality, since ec is a common factor of all terms of the series.

For the series to converge, it is necessary and sufficient that a (or at least its/realpart) be negative. If this condition is satisfied by a, then the series converges [regardless of b].

By altering the arguments a and b, the sum is transformed into another one in which r assumes not all, but only even integral values; to achieve this, one need only replace a and b by a/4 and b/4, since

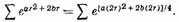
By attaching a factor, moreover, the sum can be changed to another one in which r assumes only odd values. For, since

one has

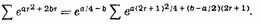
Thus one obtains the same function whether one uses all even integers for r in

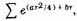
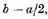
or, after replacing b by all odd integers, and then multiplies the series by the factor

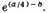
From each of these two forms of the series one obtains a new one with changed signs if b is replaced by

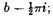
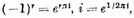
besides, the second form then has the factor i. For, since it follows that

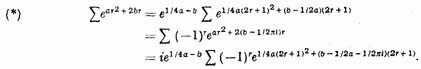
If one sets

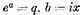
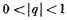
so that, by the assumption³ (which will always be tacitly made below), the above four series take on the following form:

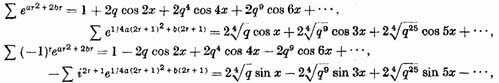
where the summations on the left side extend over all integers r.

In the scquel, these four series will be denoted by

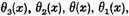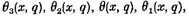
⁴ or, where necessary, by the more explicit notation so that the four theta-functions to be considered are defined by the equations⁵

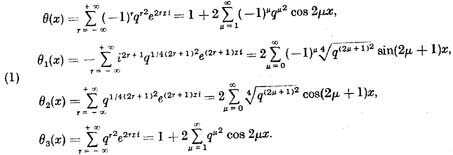
The preceding considerations show that one can pass from one function θ to the other three by changing the argument x and adjoining an exponential factor. For, by substituting in (*) q and x for a and b, respectively, one gets

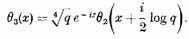
If one supplements this formula by the two following:

and the one which results from the first three,

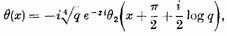
then . . . on changing the argument by

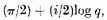
and respectively, and multiplying by a suitable exponential factor, [one obtains] the other three θ-functions from θ₂ (x). The same holds for θ(x), θ₁ (x), and θ₃ (x). The following system of formulas permits a complete overview of the relations between the θ-functions:

where

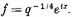
With the help of the formulas

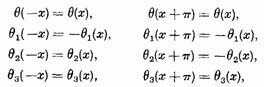
one can obtain similar formulas from (2) for the change of the argument x by

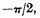
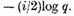
and

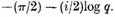
2.

The function θ₃ (x) in the form first considered is defined by the infinite exponential series

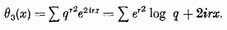
The exponent of e can be brought into the form

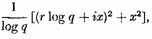
which yields the representation

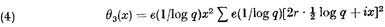
for θ₃ (x). The corresponding representation of the function θ₂ (x) is

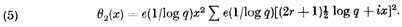
These two sums differ only in that one is taken over all (positive and negative) even integers 2r, while the other is taken over all odd integers

If several series of this kind with different values of the argument x are multiplied together, then the product can be regarded as a multiple series whose general term is an exponential whose exponent is a sum of squares. Of special interest is the case in which four such series are multiplied together, because then one obtains a sum of four squares in the exponent, to which an elementary transformation formula may be applied.

It is a well-known algebraic theorem that the sum of four squares

can always be represented in the same form in a second way. For, if one determines four new quantities by the formulas

then

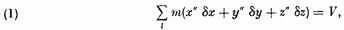
identically.

[Jacobi then expatiates on various identities and summation principles related to Waring’s problem, and hence to the considerations we have presented in Selection 42. He continues:]

Having explained these preliminaries, I return to the representations (4) and (5) of the functions

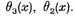
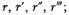
In each of these equations let four different arguments w, x, y, z be substituted for x, and at the same time let the corresponding series term be denoted by it follows that the relation

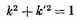
holds between k and k′.

Equations (D)⁶ show that, if one divides three of the functions

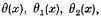
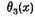
by the fourth, then two of the resulting quotients can be determined from the third by extracting square roots. Thus one obtains:

which can be expressed more elegantly: It is possible to determine an angle φ in such a way that at the same time

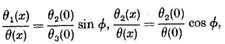
and

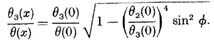
These equations, on introducing the quantities k and k′ defined above and using Legendre’s notation

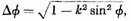
assume the form

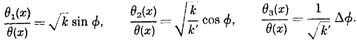
The results derived from formulas (D) and (E) can therefore be summarized in the following equations:

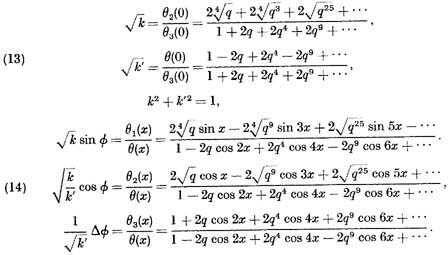
[Jacobi then explains that, of course, φ; is only determined modulo 2π by equations (14), but that if φ(x) is continuous, then it is uniquely determined by setting

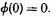
He continues:

In particular, let it be assumed that x and

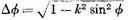
are both real; then, by (13), k and k′ are also real and less than 1, similarly, by (14), φ is real, and since by (12) the functions θ₃ (x) and θ(x) have only positive values for real values of x and q, the square root in the third equation is always to be taken with positive ?? gn.

[Jacobi then launches into several pages of formidable calculations, which he finally summarizes.]

The results obtained above may be summarized as follows:

The four θ-functions defined in §1 satisfy relations permitting the amplitude φ, the modulus k, and the complementary modulus k′ to be defined as functions of x and q by the six simultaneous equations [(13) and (14)] . . . and the condition that φ vanishes simultaneously with x. But then conversely, x can be represented as a function of and k by the equations

and one has besides

In the following, as in the Fundamenta, I shall denote the inverse function of F(φ) by am

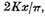
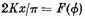
so that from there conversely follows [The paper continues with the determination of q as a function of k; this results in

Summarizing, for the amplitudes

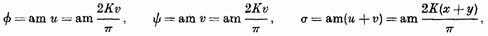
Jacobi obtains the addition theorem:

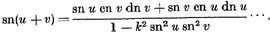
The rest contains corresponding developments for elliptic integrals of the second and third kinds.]

¹ C. G. J. Jacobi, "Theorie der elliptische Functionen aus der Theorie der Thetareihen geleitet," Werke, I, 499–538 [499–520]. This is a posthumous exposition by Borchardt of a lecture by Jacobi.

² Jacobi used v where we use r.

³ Jacobi wrote "Modul von q" where we write

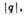
here and below.

⁴ Jacobi has here changed his notation from the Θ H, H₁, Θ of Selection 42 (and his Funda-menta Nova) to

see Whittaker and Watson, Modern Analysis, See. 21.62. Where we write θ, Jacobi and Whittaker and Watson write

⁵ Jacobi uses the simple summation symbol without indicating the limits of summation symbolically (e.g., by writing

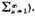
⁶ Equations (D) are the following:

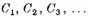
If, furthermore, one sets

then the first of equations (D) gives the curious relation