Mathematics

C. Potential Theory Develops

Green on Green’s Identities¹

§1. The function which represents the sum of all the electric particles acting on a given point divided by their respective distances from this point, has the property of giving, in a very simple form, the [attraction of] the whole electrified mass. We shall, in what follows, endeavor to discover some relations between this function and the density of the electricity in the mass or masses producing it, and apply the relations thus obtained to the theory of electricity.

Firstly, let us consider a body of any form whatever, through which the electricity is distributed according to any given law, and fixed there, and let x′, y′, z′, be the rectangular coordinates of a particle of this body, ρ′ the density of the electricity in this particle, so that dx′ dy′ dz′ being the volume of the particle, ρ′; dx′ dy′ dz′ shall be the quantity of electricity it contains; moreover, let r′ be the distance between this particle and a point p exterior to the body, and [let] V represent the sum of all the particles of electricity divided by their respective distances from this point, whose coordinates are supposed to be x, y, z, then . . .

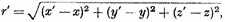
and

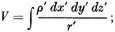
the integral comprehending every particle in the electrified mass under consideration.

Laplace has shown, in his Mécanique Céleste, that the function V has the property of satisfying the equation

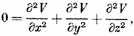
and as this equation will be incessantly recurring in what follows, we shall write it in the abridged form

the symbol being used in no other sense throughout the whole of this Essay.²

In order to prove that

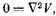
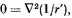
we have only to remark that by differentiation we immediately obtain and consequently each element of V substituted for V in the above equation satisfies it; hence the whole integral (being considered as the sum of all these elements) will also satisfy it. This reasoning ceases to hold good when the point p is within the body, for then, the coefficients of some of the elements which enter into V becoming infinite, it does not therefore necessarily follow that V satisfies the equation

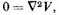
although each of its elements, considered separately, may do so.

In order to determine what

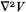
becomes for any point within the body, conceive an exceedingly small sphere whose radius is a enclosing the point p at the distance b from its center, a and b being exceedingly small quantities. 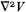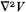Then, the value of V may be considered as composed of two parts, one due to the sphere itself, the other due to the whole mass exterior to it: but the last part evidently becomes equal to zero when substituted for V in ; we have therefore only to determine the value of for the small sphere itself, which value is known to be

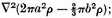
ρ being equal to the density within the sphere and consequently to the value of ρ′ at p. If now x₁ , y₁ , z₁ are the coordinates of the center of the sphere, we have

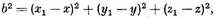
and consequently

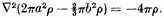
Hence, throughout the interior of the mass,

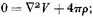
of which, the equation

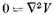
for any point exterior to the body is a particular case, seeing that here

Let now q be any line terminating in the point p, supposed without the body, then −∂V/∂q = the force tending to impel a particle of positive electricity in the direction of q, and tending to increase it. This is evident, because each of the elements . . . substituted for V in −∂V/∂q will give the force arising from this element in the direction tending to increase q, and consequently −∂V/∂q will give the sum of all the forces due to every clement of V, or the total force acting on p in the same direction. In order to show that this will still hold good, although the point p be within the body, conceive the value of V to be divided into two parts as before, and moreover let p be at the surface of the small sphere or

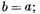
then the force exerted by this small sphere will be expressed by

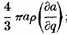
da being the increment of the radius a, corresponding to the increment dq of q, which force evidently vanishes when

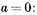
we need therefore have regard only to the part due to the mass exterior to the sphere, and this is evidently equal to

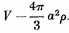
But as the first differentials of this quantity are the same as those of V when z is made to vanish, it is clear that whether the point p be within or without the mass, the force acting upon it in the direction of increasing q is always given by −∂V/∂q.

Although in what precedes we have spoken of one body only, the reasoning . . . is general, and will apply equally to a system of any number of bodies whatever, in those cases even, where there is a finite quantity of electricity spread over their surfaces, and it is evident that we shall have for a point p in the interior of any one of these bodies

Moreover, the force tending to [lengthen] a line q ending in any point p within or without the bodies, will be likewise given by −∂V/∂q; the function V representing the sum of all the electric particles in the system divided by their respective distances from p. As this function, which gives [so simply] the forces by which a particle p of electricity, anyhow situated, is impelled, will recur very frequently in what follows, we have ventured to call it the potential function belonging to the system, and it will evidently be a function of the coordinates of the particle p under consideration.

§2. It has been long known from experience, that whenever the electric fluid is in a state of equilibrium in any system whatever of perfectly conducting bodies, the whole of the electric fluid will be carried to the surface of those bodies, without the smallest portion of electricity remaining in their interior: but I do not know that this has ever been shown to be a necessary consequence of the law of electric repulsion, which is found to take place in nature. This however may be shown to be the case for every imaginable system of conducting bodies, and is an immediate consequence of what has preceded. For let x, y, z be the rectangular coordinates of any particle p in the interior of one of the bodies; then will −∂V/∂x be the force with which p is impelled in the direction of the coordinate x, and tending to increase it. In the same way, −∂V/∂y and −∂V/∂z will be the forces in y and z, and since the fluid is in equilibrium all these forces are equal to zero: hence

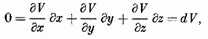
which equation being integrated gives

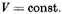
This value of V being substituted in the equation (1) of the preceding number gives

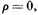
and consequently shows that the density of the electricity at any point in the interior of any body in the system is equal to zero.

The same equation (1) will give the value of ρ the density of the electricity in the interior of any of the bodies, when there are not perfect conductors, provided we can ascertain the value of the potential function V in their interior.

§3. Before proceeding to make known some relations which exist between the density of the electric fluid at the surfaces of bodies, and the corresponding values of the potential functions within and without those surfaces, the electric fluid being confined to them alone, we shall in the first place lay down a general theorem which will afterwards be very useful to us. This theorem may be thus enunciated:

Let U and V be two continuous functions of the rectangular coordinates x, y, z, whose differential coefficients do not become infinite at any point within a solid body of any form whatever; then will

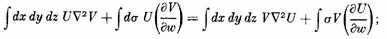
the triple integrals extending over the whole interior of the body, and those relative to dσ, over its surface, of which dσ represents an element, dw being an infinitely small line perpendicular to the surface and measured from this surface towards the interior of the body.

To prove this let us consider the triple integral

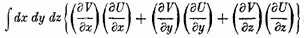
The method of integration by parts reduces this to

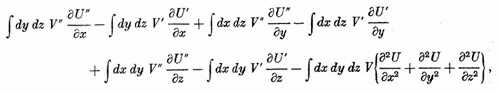
the accents over the quantities indicating, as usual, the values of those quantities at the limits of the integral, which in the present case are on the surface of the body, over whose interior the triple integrals are supposed to extend.

Let us now consider the part

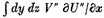
due to the greater values of x. It is easy to see since dw is everywhere perpendicular to the surface of the solid, that if dσ″ be the element of this surface corresponding to dy dz, we shall have

and hence by substitution

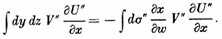
In like manner it is seen that in the part

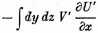
due to the smaller values of x, we shall have

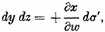
and consequently,

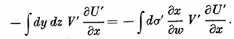
Then, since the sum of the elements represented by dσ′, together with those represented by dσ″, constitutes the whole surface of the body, we have by adding these two parts

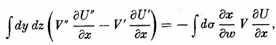
where the integral relative to dσ is supposed to extend over the whole surface, and dx to be the increment of x corresponding to the increment dw.

In precisely the same way we have

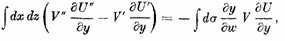
and

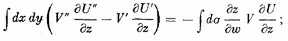
therefore, the sum of all the double integrals in the expression before given will be obtained by adding together the three parts just found; we shall thus have

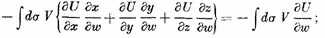
where V and ∂U/∂w represent the values at the surface of the body. Hence, the integral

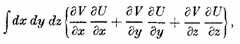
by using the characteristic

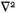
in order to abridge the expression, becomes

Since the value of the integral just given remains unchanged when we substitute V for U and reciprocally, it is clear that it will also be expressed by

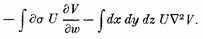
Hence, if we equate these two expressions of the same quantity, after having changed their signs, we shall have [the identity]³

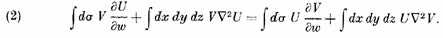
Thus the theorem appears to be completely established, whatever may be . . . the functions U and V.

In our enunciation of the theorem, we have supposed the differentials of U and V to be finite within the body under consideration, a condition, the necessity of which does not appear explicitly in the demonstration, but which is understood in the method of integration by parts there employed.

In order to show more clearly the necessity of this condition, we will now determine the modification which the formula must undergo, when one of the functions, U for example, becomes infinite within the body; and let us suppose it to do so in one point p′ only; moreover, infinitely near this point let U be sensibly equal to 1/r, r being the distance between the point p′ and the element dx dy dz. Then if we suppose an infinitely small sphere whose radius is a to be described round p′, it is clear that our theorem is applicable to the whole of the body exterior to this sphere, and since

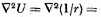
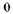
within the sphere, it is evident the triple integrals may still be supposed to extend over the whole body, as the greatest error that this supposition can induce, is a quantity of the order a². Moreover, the part of ∫ dσ U ∂V/∂w due to the surface of the small sphere is only an infinitely small quantity of the order a; there only remains therefore to consider the part of ∫ dσ V ∂U/∂w due to this same surface, which, since we have here

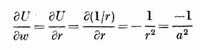
becomes −4πV′ when the radius a is supposed to vanish. Thus, the equation (2) becomes

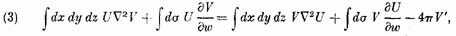
where, as in the former equation, the triple integrals extend over the whole volume of the body, and those relative to dσ over its exterior surface, V′ being the value of V at the point p′.

[Likewise,] if the function V . . . becomes infinite for any point p″ within the body, and is moreover sensibly equal to 1/r′ infinitely near this point, as U is infinitely near to the point p′, it is evident from what has preceded that we shall have

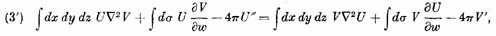
the integrals being taken as before, and U″ representing the value of U at the point p″ where V becomes infinite. The same process will evidently apply, however great may be the number of similar points belonging to the functions U and V.

For abridgment, we shall in what follows call those singular values of a given function, where its differential coefficients become infinite, and the condition originally imposed upon U and V will be expressed by saying that neither of them has any singular values within the solid body under consideration.

§4. We will now proceed to determine some relations existing between the density of the electric fluid at the surface of a body, and the potential functions thence arising within and without this surface. For this, let ρdσ be the quantity of electricity of an clement dσ of the surface, and V, the value of the potential function for any point p within it, of which the coordinates are x, y, z. Then, if V′ be the value of this function for any other point p′ exterior to this surface, we shall have

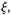η, ζ being the coordinates of dσ, and

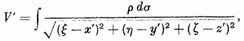
the integrals relative to dσ extending over the whole surface of the body.

It might appear at first view, that to obtain the value of V′ from that of V, we should merely have to change x, y, z into x′, y′, z′: but this is by no means the case; for the form of the potential function changes suddenly, in passing from the space within to that without the surface. Of this, we may give a very simple example, by supposing the surface to be a sphere whose radius is a and center at the origin of the coordinates; then, if the density ρ be constant, we shall have

and

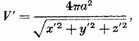
which are essentially distinct functions.

With respect to the functions V and V′ in the general case, it is clear that each of them will satisfy Laplace’s equation, and consequently

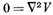
and

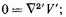
moreover, neither of them will have singular values, for any point of the spaces to which they respectively belong; and at the surface itself we shall have

the horizontal bars over the quantities indicating that they belong to the surface. At an infinite distance from this surface, we shall likewise have

We will now show that if any two functions whatever are taken, satisfying these conditions, [there is] always one and only one value of ρ;, which will produce them for corresponding potential functions. For this we may remark that equation (3), art. 3, being applied to the space within the body, becomes, by making

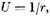
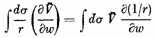
since

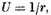
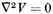
has but one singular point, viz. p; and we have also and 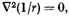r being the distance between the point p to which V belongs and the element dσ.

If now we conceive a surface enclosing the body at an infinite distance from it, we shall have, by applying the formula (2) of the same article to the space between the surface of the body and this imaginary exterior surface (seeing that here

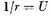
has no singular value)

since the part due to the infinite surface may be neglected, because V′ is there equal to zero. In this last equation, it is evident that w′ is measured from the surface into the exterior space, and hence

which equation reduces the sum of the two just given to

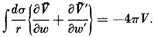
In exactly the same way, for the point p′ exterior to the surface, we shall obtain

Hence . . . there exists a value of ρ, viz.

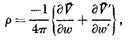
which will give V and V′ for the two potential functions, V within and V′ without the surface.

Again,

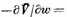
force with which a particle of positive electricity p, placed within the surface and infinitely near it, is impelled in the direction dn perpendicular to this surface, and directed inwards; and −∂V′/∂w′ expresses the force with which a similar particle p′ placed without this surface, on the same normal with p, and also infinitely near it, is impelled outwards in the direction of this normal. But the sum of these two forces is equal to double the force that an infinite plane would exert upon p, supposing it uniformly covered with electricity of the same density as at the foot of the normal on which p is; and this last force is easily shown to be expressed by 2πρ, hence by equating

on the surface, and consequently there is only one value of ρ which can produce V and V′ as corresponding potential functions.

Although in what precedes, we have considered the surface of one body only, the same arguments apply, [to more bodies, no matter] how great . . . their number; for the potential functions V and V′ would still be given by the formulas

the only difference would be that the integrations must now extend over the surface of all the bodies, and that the number of functions represented by V would be equal to the number of the bodies, one for each. In this case, if there were given a value of V for each body, together with V′ belonging to the exterior space; and moreover, if these functions satisfied the above-mentioned conditions, it would always be possible to determine the density on the surface of each body, so as to produce these values as potential functions, and there would be but one density, viz. that given by

which could do so: ρ,

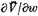
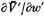
, and belonging to a point on the surface of any of these bodies.

§5. From what has been before established (art. 3), it is easy to prove that when the value of the potential function 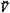is given on any closed surface, there is but one function which can satisfy at the same time the equation

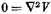
and the condition that V shall have no singular values within this surface. For equation (3), art. 3, becomes by supposing

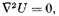
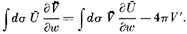
In this equation, U is supposed to have only one singular value within the surface, viz. at the point p′, and, infinitely near to this point, to be sensibly equal to 1/r; r being the distance from p′. If now we had a value of U which, besides satisfying the above-written conditions, was equal to zero at the surface itself, we should have

and this equation would become

which shows that V′, the value of V at the point p′, is given, when 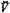, its value at the surface, is known.

To convince ourselves that there does exist such a function as we have supposed U to be, conceive the surface to be a perfect conductor put in communication with the earth, and a unit of positive electricity to be concentrated in the point p′; then the total potential function arising from p′ and from the electricity it will induce upon the surface will be the required value of U. For, in consequence of the communication established between the conducting surface and the earth, the total potential function at this surface must be constant, and equal to that of the earth itself, i.e., to zero (seeing that in this state they form but one conducting body). Taking, therefore, this total potential function for U, we have evidently

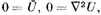
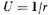
and for those parts infinitely near to p′. As, moreover, this function has no other singular points within the surface, it evidently possesses all the properties assigned to U in the preceding proof.

Again, since we have evidently

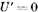
, for all the space exterior to the surface, equation (4), art. 4, gives

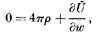
where ρ is the density of the electricity induced on the surface by the action of a unit of electricity concentrated in the point p′. Thus, equation (5) of this article becomes

This equation is remarkable on account of its simplicity and singularity, seeing that it gives the value of the potential for any point p′, within the surface, when 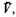its value at the surface itself, is known, together with ρ, the density that a unit of electricity concentrated in p′ would induce on this surface, if it conducted electricity perfectly, and were put in communication with the earth.

Having thus proved that V′, the value of the potential function V at any point p′ within the surface, is [determined] provided its value is known at this surface, we will now show that whatever the value of 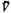may be, the general value of V deduced from it by the formula just given shall satisfy the equation

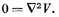
For, the value of V at any point p whose coordinates are x, y, z, deduced from the assumed value of

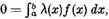
by the above-written formula, is

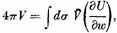
U being the total potential function within the surface, arising from a unit of electricity concentrated in the point p, and the electricity induced on the surface itself by its action. Then, since 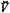is evidently independent of x, y, z, we immediately deduce

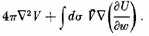
Now the general value of U will depend upon the position of the point p producing it, and upon that of any other point p′ whose coordinates are x′, y′, z′, to which it is referred, and will consequently be a function of the six quantities, x, y, z, x′, y′, z′. But we may conceive U to be divided into two parts, one = 1/r (r being the distance pp′) arising from the electricity in p, the other, due to the electricity induced on the surface by the action of p, and which we shall call U′. Then since U′ has no singular values within the surface, we may deduce its general value from that at the surface, by a formula similar to the one just given. Thus

where U′ is the total potential function which would be produced by a unit of electricity in p′, and therefore,

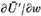
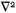
is independent of the coordinates x, y, z, of p, to which refers.

Hence

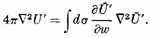
We have before supposed

and as

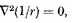
we immediately obtain

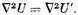
Again, since we have at the surface itself

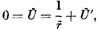
being the distance between p and the element dσ, we hence deduce

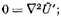
this substituted in the general value of

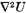
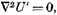
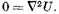
before given, there arises and consequently The result just obtained being general, and applicable to any point p″ within the surface, gives immediately

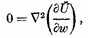
and we have by substituting in the equation determining

,

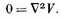
In a preceding part of this article, we have obtained the equation

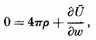
which combined with

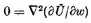
gives

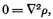
and therefore the density ρ induced on any element dσ, which is evidently a function of the coordinates x, y, z of p, is also [harmonic]; it is moreover evident that ρ can never become infinite when p is within the surface.

It now remains to prove that the formula

[gives a function V within the surface which always tends to the limit 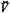as the surface is approached].⁴

For this, suppose the point p to approach infinitely near the surface; then it is clear that the value of ρ, the density of the electricity induced by p, will be insensible, except for those parts infinitely near to p, and in these parts it is easy to see that the value of ρ will be independent of the form of the surface, and depend only oil the distance p, dσ. But we shall afterwards show (art. 10), that when this surface is a sphere of any radius whatever, the value of ρ is

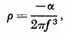
α being the shortest distance between p and the surface, and f representing the distance p, dσ. This expression will give an idea of the rapidity with which ρ decreases, in passing from the infinitely small portion of the surface in the immediate vicinity of p, to any other part situate at a finite distance from it . . . . It is also evident that the function V, determined by the above-written formula, will have no singular values within the surface under consideration.

[The author continues by proving the formula expressing the symmetry of Green’s function.]

¹ For a spate of references, see the article by H. Burkhardt and W. Mayer in Enz. Math. Wiss., IIA, 7b, where reference is made to Burkhardt’s 1800-page article in the Jahresb. Deut. Math. Ver. 10 (1904–08).

² See E. T. Whittaker, History of the Theories of the Aether and Electricity, 2d ed., p. 65; see, however, the next note. In 1744, Euler had called the strain energy of an elastica its vis potentialis.

³ Klein, Entwicklung, p. 22; cf. also footnote 1.

⁴ As is noted in Kellogg, Potential Theory, p. 38, precursors of the Divergence Theorem are found in Lagrange, Oeuvres, I, 263, and Gauss, Werke, V, 5–7.

¹ G. Green, "An essay on the application of mathematical analysis to the theories of electricity and magnetism," Papers, pp. 1–115 [10–13; 19–41].

² Green uses "δ" for "

"

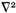
³ This is Green’s Second Identity.

⁴ Green writes "shall always give

for any point within the surface and infinitely near it, whatever may be the assumed value of ." He also writes (ρ) where we have written ρ.