|
|
A Source Book in Mathematics, 1200-1800
Contents:
Show Summary
Hide Summary
Historical SummaryRené DescartesIn his Géométrie of 1637, Descartes applied his reformed algebra (see Selection II.7) to the geometry of the Ancients In Book I he applies his coordinate method to Pappus’ problem (see the previous Selection). The required locus can then be expressed by a relation between two variables which he denotes by x and y and in which we recognize oblique "Cartesian" corodinates."Since there is only one condition to be expressed . . . we may give any value we please to either the one or the other of the unknown quantities x or y, and find the value of the other from this equation. It is evident that when no more than five lines are given, the quantity x, which is not used to express the first of the lines, can never be of degree [dimension] higher than the second. Assigning thus a given value to y, we have only [il ne restera que ], and therefore the quantity x can be found with ruler and compasses, by a method already explained" (Smith and Latham, The geometry of René Descartes, p. 34; see Selection II.8).Then, in Book II, after a classification of the problems of geometry into plane, solid, and linear ones (according to Pappus; see Selection III.2). Descartes suggests that a further classification of these "linear" curves is desirable, but that the classical distinction between geometrical and mechanical curves does not seem justified, since circles and straight lines can also be considered instruments [machines]. He then discusses some of these mechanical ways of describing a curve, and gives ((pp. 49–55 of the Smith-Latham translation) the following example of his coordinate method:Then Descartes continues with his solution of the problem of Pappus, which leads him to the consideration of conic sections and other curves with several types of equations, such asHere also is Descartes’s method of finding the equation of a normal to a curve. This method was in a sense opposed to that of Fermat, whose method was based on finding first the equation of a tangent to a curve (see Selection IV.8) and thus came close to the idea of a derivative.Book III of the Géométrie contains algebra; see Selection II.7.
Mathematics
5 DESCARTES.
The Equation of a Curve
I wish to know the genre1 of the curve EC [Fig. 1], which I imagine to be described by the intersection of the ruler GL and the rectilinear plane figure CNKL, whose side KN is produced indefinitely in the direction of C, and which,
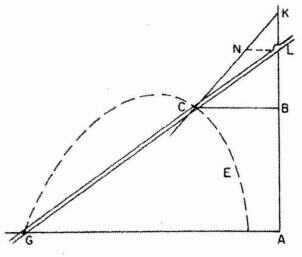 Fig. 1 Fig. 1
being moved in the same plane in such a way that its side KL always coincides with some part of the line BA (produced in both directions), imparts to the ruler GL a rotary motion about G (the ruler being so connected to the figure CNKL that it always passes through L).2 If I wish to find out to what genre this curve belongs, I choose a straight line, as AB, to which to refer all its points, and in AB I choose a point like A at which to begin the calculation. I say that I choose the one and the other, because we are free to choose them as we like, for while it is necessary to use care in the choice in order to make the equation as short and simple as possible, yet no matter what line I should take instead of AB the curve would always prove to be of the same genre, a fact easily demonstrated.
Then I take on the curve an arbitrary point, as C, at which I will suppose that the instrument to describe the curve is applied. Then I draw through C the line CB parallel to GA. Since CB and BA are unknown and indeterminate quantities, I shall call one of them y and the other x. But in order to find the relation between these quantities I consider also the known quantities which determine the description of the curve, as GA, which I shall call a; KL, which I shall call b; and NL, parallel to GA, which I shall call c. Then I say that as NL is to LK, or as c is to b, so CB, or y, is to BK, which is therefore equal to
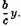
Then BL is equal to
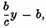
and AL is equal to
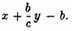
Moreover, as CB is to LB, that is, as y is to
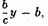
so AG or a is to LA or
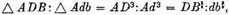
Multiplying the second by the third, we get
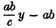
equal to
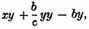
which is obtained by multiplying the first by the last. Therefore, the required equation is
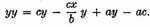
From this equation we see that the curve EC belongs to the first genre, it being, in fact, a hyperbola.
If in the instrument used to describe the curve we substitute for the straight line CNK this hyperbola or some other curve of the first genre lying in the plane CNKL, the intersection of this curve with the ruler GL will describe, instead of the hyperbola EC, another curve, which will be of the second genre.
Thus, if CNK be a circle having its center at L, then we shall describe the first Conchoid of the Ancients,3 while if we use a parabola having KB as diameter we shall describe the curve which, as I have already said, is the first and simplest of the curves required in the problem of Pappus, that is, the one which furnishes the solution when five lines are given in position.4
1 Earlier in Book II, Descartes has defined the genre of a curve. In our terms: If an algebraic curve has degree

its genre is n. This terminology may have boon inspired by the problem of Pappus. Newton (see Selection III.8) translates genre by genus.
2 The instrument thus consists of three parts: (1) a ruler AK of indefinite length, fixed in the plane; (2) a ruler GL, also of indefinite length, passing through a pivot G in this plane (but not on AK); and (3) a triangle LNK, KN indefinitely extended toward KC, to which the ruler GL is connected at L so as to make the triangle slide with its side KL along AB.
3 Pappus mentions four types of conchoid (shell curves); the first is the one we still call a conchoid, in polar coordinates
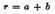
sec θ. It is a curve of the third degree, therefore of the second genre of Descartes.
4 This is also a curve of the second genre.
Contents:
Chicago:
René Descartes, "The Equation of a Curve," A Source Book in Mathematics, 1200-1800, trans. Latham in A Source Book in Mathematics, 1200-1800, ed. D. J. Struik (Princeton: Princeton University Press, 1969, 1986), 155–157. Original Sources, accessed October 17, 2025, http://originalsources.com/Document.aspx?DocID=8W643NYWAAQU23F.
MLA:
Descartes, René. "The Equation of a Curve." A Source Book in Mathematics, 1200-1800, translted by Latham, in A Source Book in Mathematics, 1200-1800, edited by D. J. Struik, Princeton, Princeton University Press, 1969, 1986, pp. 155–157. Original Sources. 17 Oct. 2025. http://originalsources.com/Document.aspx?DocID=8W643NYWAAQU23F.
Harvard:
Descartes, R, 'The Equation of a Curve' in A Source Book in Mathematics, 1200-1800, trans. . cited in 1969, 1986, A Source Book in Mathematics, 1200-1800, ed. , Princeton University Press, Princeton, pp.155–157. Original Sources, retrieved 17 October 2025, from http://originalsources.com/Document.aspx?DocID=8W643NYWAAQU23F.
|