|
|
A Source Book in Physics
Contents:
Show Summary
Hide Summary
General SummaryAn explanation which Descartes gives of the sending of the different colors in different directions, based upon his speculations on the nature and structure of the medium which transmits light through bodies, is omitted. Descartes then presents his geometrical method of calculating the deviations of the rays of light which fall on different selected points on the surface of a spherical drop, and gives tables of these deviations which illustrate the preceding statements.
Historical SummaryThe following extract is from Descartes’ treatise, Les Méteores, which was also published in 1637 as a supplement to the Discours de la Methode. In it Descartes explains the position of the primary and secondary rainbows. He was not able to account for the colors of the bows in any satisfactory way. This was first done by Newton.
THE RAINBOW
The rainbow is such a remarkable natural wonder and its cause has been so zealously sought by able men and is so little understood, that I thought that there was nothing I could choose which is better suited to show how, by the method which I employ, we can arrive at knowledge which those whose writings we possess have not had. In the first place, considering that this bow appears not only in the sky, but also in the air near us, wherever there are drops of water illuminated by the sun, as we can see in certain fountains, I readily decided that it arose only from the way in which the rays of light act on these drops and pass from them to our eyes. Further, knowing that the drops are round, as has been formerly proved, and seeing that whether they are larger or smaller, the appearance of the bow is not changed in any way, I had the idea of making a very large one, so that I could examine it better. For this purpose I filled with water a large glass phial, perfectly spherical in shape and very transparent, and then found that if the sunlight came, for example, from the part of the sky which is marked AFZ (Fig. 52), and my eye was at the point E, when I put the globe in the position BCD, its part D appeared all red, and much more brilliant than the rest of it; and that whether I approached it, or receded from it, or put it on my right or my left, or even turned it round about my head, provided that the line DE always made an angle of about forty-two degrees with the line EM, which we are to think of as drawn from the center of the sun to the eye, the part D appeared always similarly red; but that as soon as I made this angle DEM even a little larger, the red color disappeared; and if I made the angle a little smaller, the color did not disappear so all at once, but divided itself first as if into two parts, less brilliant, and in which I could see yellow, blue, and other colors. Next, when I looked at that part of the globe which is marked K, I saw that, if I made the angle KEM about fifty-two degrees, the part K appeared also of a red color, but not so bright as that at D; and if I made the angle a little larger, there appeared other less brilliant colors, but if I made it even a little smaller, or much larger, no colors at all appeared. From which I clearly perceived that if all the air which is near M is filled with such globes, or instead of them with drops of water, there ought to appear a bright red point in every one of the drops so placed that the lines drawn from them to the eye at E make an angle of about forty-two degrees with the line EM, as I suppose those do which are marked R; and that if we look at all these points together, without any consideration of their exact position except of the angle at which they are viewed, they should appear as a continuous circle of a red color; and that something similar ought to appear at the points marked S and T, the lines drawn from which to E make more acute angles with EM, where there will be circles of less brilliant colors. This constitutes the first and principal rainbow. And further if the angle MEK is fifty-two degrees, there should appear a red circle in the drops marked X; and other circles of less brilliant colors in the drops marked Y. This constitutes the second and less important rainbow. And finally in all the other drops marked V no colors at all should appear. When I examined more particularly, in the globe BCD, what it was which made the part D appear red, I found that it was the rays of the sun which, coming from A to B, bend on entering the water at the point B, and pass to C, where they are reflected to D, and bending there again as they pass out of the water, proceed to the point E; for when I put an opaque body or screen in any part of the lines AB, BC, CD, or DE, the red color disappeared. And although I covered all the globe except the two points B and D, and set up screens everywhere else, provided that I did not interfere with the rays ABCDE, the red never failed to appear. Then when I sought also for the cause of the red that appeared at K, I found that it was the rays which come from F to G, where they bend towards H, and at H are reflected to I, where they are again reflected to K, and finally bend at K and proceed to E. So that the first bow is caused by the rays which come to the eye after two refractions and one reflection, and the second by other rays which reach the eye only after two refractions and two reflections, so that it does not appear so often as the first one.
 FIG. 52. FIG. 52.
But the principal difficulty still remained, which was to determine why, since there are many other rays which can reach the eye after two refractions and one or two reflections when the globe is in some other position, it is only those of which I have spoken which exhibit the colors. And to answer that question I asked myself if there were not some other method of making the colors appear, so that by a comparison of the two I might better determine the reason for them. Then remembering that a prism or triangle of glass shows similar colors, I considered such a prism as that represented at MNP (Fig. 53), whose two surfaces MN and NP are plane and inclined to each other, at an angle of 30 or 40 degrees, so that if the rays of the sun ABC traverse MN perpendicularly or almost perpendicularly, so that they experience no appreciable refraction, they will experience a considerable refraction as they pass out through NP. And when I covered one of these surfaces with a screen, in which there was a small opening DE, I observed that the rays which pass through this opening and are received on a white cloth or sheet of paper, show all the colors of the rainbow; and that the red always appears at F and the blue or violet at H. From which I learned, in the first place, that the curvature of the surface of the drops of water is not necessary for the production of the colors; for the surfaces of the crystal are plane; and that the size of the angle at which the colors appear is not important, since that can be changed without changing the colors; and even if we make the rays which go to F bend sometimes more and sometimes less than those which go to H, they still never fail to give red, and those which go to H to give blue; nor is reflection necessary, for there is none; nor finally the plurality of refractions, for in this case there is only one. I decided however that at least one refraction is necessary, and one the effect of which is not destroyed by another contrary one; for experiment shows that if the surfaces MN and NP are parallel, the rays which are bent at the one surface return to their original direction at the other, and produce no colors. I had no doubt that light was necessary, for without it we should see nothing. And further I observed that a shadow or a limitation of the light was necessary; for if we remove the screen on NP the colors FGH no longer appear; and if we make the opening DE large enough, the red, the orange, and the yellow which go to F do not move farther out, nor do the green, the blue, and the violet, which go to H, but all the rest of the space between them at G remains white.
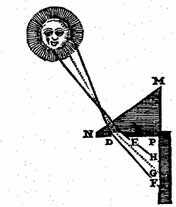 FIG. 53. FIG. 53.
However I was in doubt whether the colors of the rainbow are produced in the same way as they are in the crystal MNP; for I saw no shadow there to limit the light, and did not understand why the colors appeared only at certain angles; until I took my pen and made an accurate calculation of the paths of the rays which fall on the different points of a globe of water, to determine at what angles, after two refractions and one or two reflections they will come to the eye, and then I found that after one reflection and two refractions there are many more rays which can be seen at an angle of from forty-one to forty-two degrees than at any smaller angle; and that there are none which can be seen at a larger angle. I found also that, after two reflections and two refractions, there are many more rays which come to the eye at an angle of from fifty-one to fifty-two degrees than at any larger angle, and none which come at a smaller angle. Thus there is a shadow on one side and the other, which limits the light which, after having passed through an infinity of drops of rain illuminated by the sun, comes to the eye, at the angle of forty-two degrees or a little less, and thus causes the first and principal rainbow; and there is also a shadow which limits the light which comes at the angle of fifty-one degrees or a little greater, and causes the exterior bow; for to receive no rays of light in the eye, or to receive notably less light from an object than from another one which is near it, is to see a shadow. This shows clearly that the colors of these rainbows are produced by the same cause as that which produces them when we use the crystal MNP, and that the semi-diameter of the interior bow should not be greater than forty-two degrees or that of the exterior bow less than fifty-one degrees; and finally that the former should be more sharply limited at its outer edge than at its inner edge; and exactly the contrary with the latter, as is verified by observation.
Contents:
Chicago:
René Descartes, "The Rainbow," A Source Book in Physics in A Source Book in Physics, ed. William Frances Magie (Cambridge: Harvard University Press, 1935), 273–277. Original Sources, accessed September 18, 2025, http://originalsources.com/Document.aspx?DocID=72YN4GNHFL57VRE.
MLA:
Descartes, René. "The Rainbow." A Source Book in Physics, in A Source Book in Physics, edited by William Frances Magie, Cambridge, Harvard University Press, 1935, pp. 273–277. Original Sources. 18 Sep. 2025. http://originalsources.com/Document.aspx?DocID=72YN4GNHFL57VRE.
Harvard:
Descartes, R, 'The Rainbow' in A Source Book in Physics. cited in 1935, A Source Book in Physics, ed. , Harvard University Press, Cambridge, pp.273–277. Original Sources, retrieved 18 September 2025, from http://originalsources.com/Document.aspx?DocID=72YN4GNHFL57VRE.
|